Define Derivatives Math

That is the amount by which a function is changing at one given point.

Define derivatives math. The graph of a derivative of a function f x is related to the graph of f x. Note that we replaced all the a s in 1 1 with x s to acknowledge the fact that the derivative is really a function as well. The derivative of a function describes the function s instantaneous rate of change at a certain point. Historically there was and maybe still is a fight betweenmathematicians which of the two illustrates the concept of thederivative best and which one is more useful.

Simplify x2 and x2 cancel. In mathematics particularly in differential calculus the derivative is a way to show instantaneous rate of change. The definition of the derivative can beapproached in two different ways. One is geometrical as a slopeof a curve and the other one is physical as a rate of change.

The process of finding the derivative is called differentiation. Together with the integral derivative occupies a central place in calculus. 2x δx δx 2 δx. For functions that act on the real numbers it is the slope of the tangent line at a point on a graph.

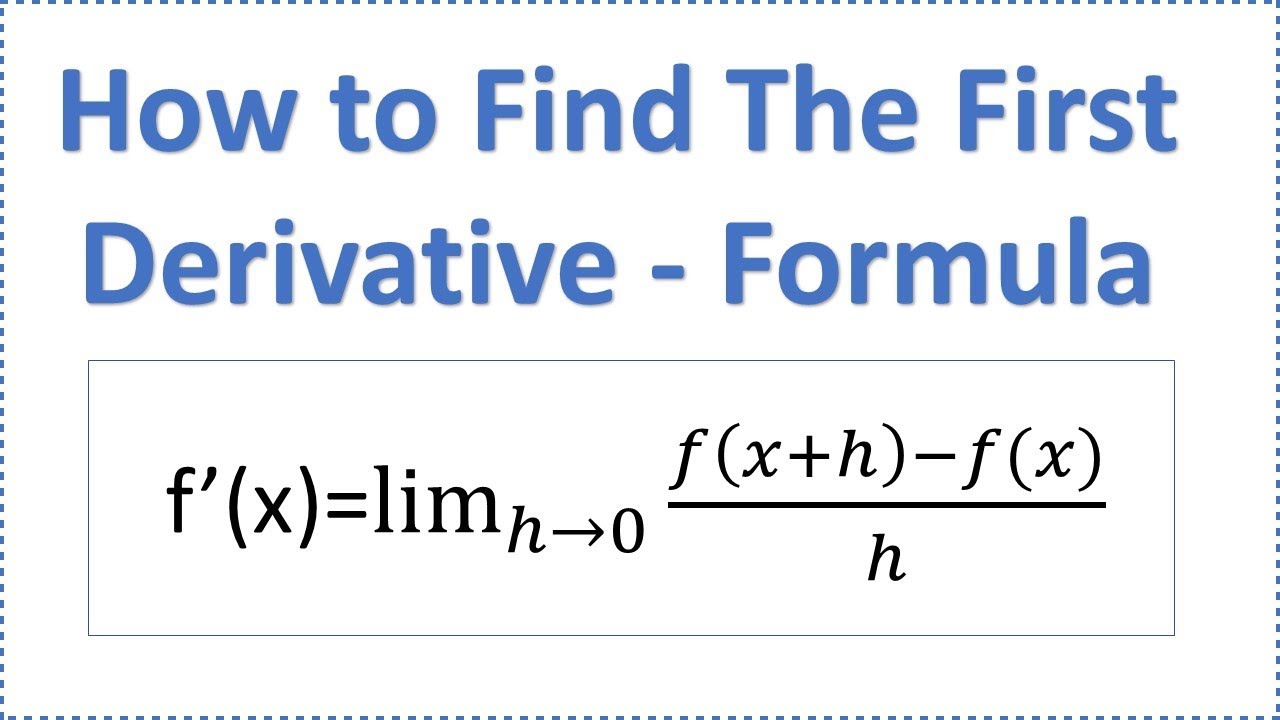
The d is not a variable and therefore cannot be cance. The derivative of x2 is 2x. The derivative of f x f x with respect to x is the function f x f x and is defined as f x lim h 0 f x h f x h 2 2 f x lim h 0. Another common interpretation is that the derivative gives us the slope of the line tangent to the function s graph at that point.

The concept of derivativeis at the core of calculus andmodern mathematics. The jacobian matrix reduces to a 1 1 matrix whose only entry is the derivative f x. The derivative of a function f x is the function whose value at x is f x. Definition of the derivative.

Put in f x δx and f x. The derivative is often written as d y d x displaystyle tfrac dy dx dy over dx meaning the difference in y divided by the difference in x. That is if f is a real valued function of a real variable then the total derivative exists if and only if the usual derivative exists. X2 2x δx δx 2 x2 δx.

In other words the slope at x is 2x. Where x has a tangent line with negative slope f x 0. The definition of the total derivative subsumes the definition of the derivative in one variable. Where f x has a tangent line with positive slope f x 0.

Simplify more divide through by δx. Learn about a bunch of very useful rules like the power product and quotient rules that help us find. The derivative of a function is one of the basic concepts of mathematics. Then as δx heads towards 0 we get.

Learn how we define the derivative using limits. 2x δx.