How To Add Vectors Mathematically

In this section we will add the same vectors mathematically. Vectors can be added using the nose to tail method or head to tail method. X horizontal component magnitude cos θ y vertical component magnitude sin θ.

probability and statistics math problems is 15 a prime number math z denotes in maths stem and leaf plot worksheet math maximum meaning in math polynomial function example math formula for rectangular prism math bar notation math

Vectors Cazoom Maths Worksheets Transformations Math Studying Gcse

13 Adding Two Vectors Graphically In Physics Vector Sum Resultant Youtube

Intro To Vectors Article Khan Academy

How To Work With Vectors Exam Question Youtube

Adding Vectors Algebraically Youtube

Vectors Magnitude Of A Vector 3d Examsolutions Youtube

17 Calculating Vector Components In Physics Part 1 Component Form Of A Youtube

Addition Of Vectors By Means Components Physics Youtube

The sum of 2 4 and 1 5 is 2 1 4 5 which is 3 9.

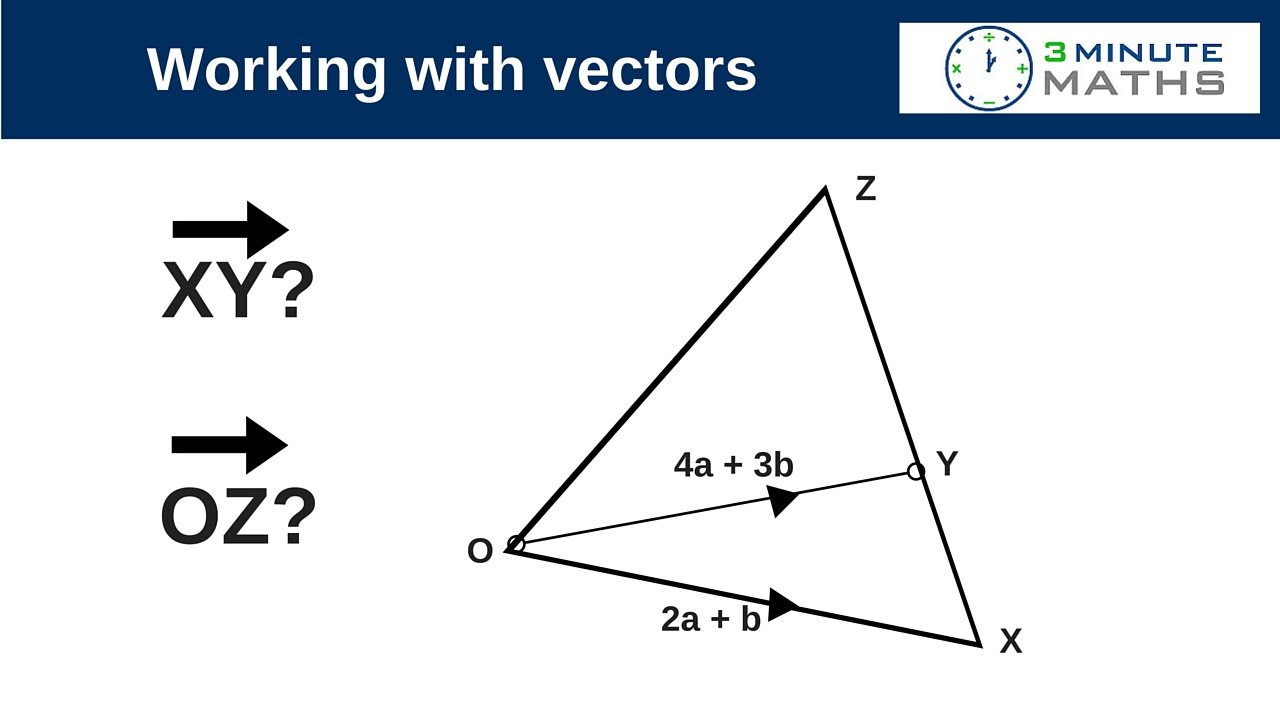
How to add vectors mathematically. Here s a concrete example. By positioning its tail at the origin. To do this we first must resolve each vector into its horizontal and vertical components. There s also a nice graphical way to add vectors and the two ways will always result in the same vector.

We see from the graphic on the left that. Note that as with scalars addition of vectors is commutative but subtraction is not. Alternatively the tail of vector a can be joined to the nose of vector b. Graphically we add two vectors a and b by positioning the tail of b at the head of a and then creating a new vector starting from the tail of a and ending at the head of b.

The coordinates of this new vector are determined in the same way as before. To add the vectors x y and x y we add the corresponding components from each vector. Two vectors a and b represented by the line segments can be added by joining the tail of vector b to the nose of vector a.