Mathematical Induction 1 2 2 2 3 2 N 2 Formula

Therefore by the principle of mathematical induction 1 3 2 3 3 3.

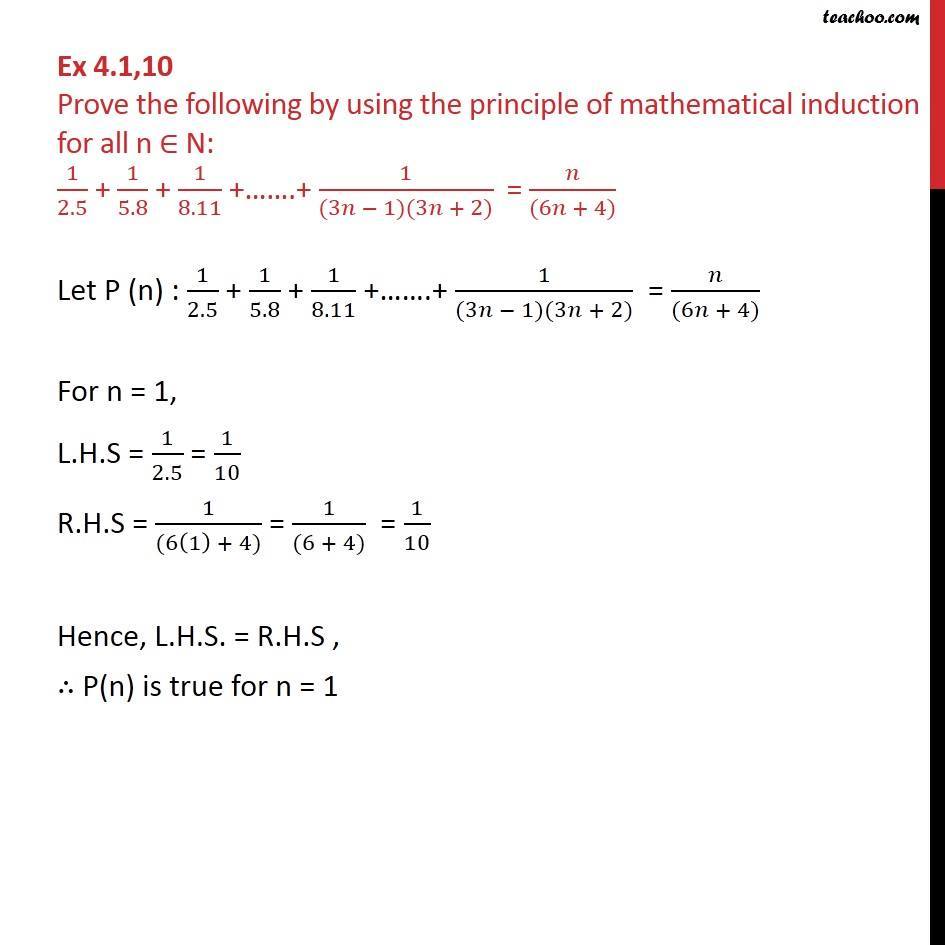
Mathematical induction 1 2 2 2 3 2 n 2 formula. Prove the following by using the principle of mathematical induction for all n n. If x 1then. Show that if any one is true then the next one is true. N n n 1 2 for n n is a natural number step 1.

Using the method of color blue proof by induction this involves the following steps prove true for some value say n 1 assume the result is true for n k. Showing that the formula holds true for n k 1. N n n 1 2 step. Prove that for any natural number n 2 1 2 2 1 3 1 n 1.

Induction method involves two steps one that the statement is true for n 1 and say n 2. 1 2 2 22 3 23 n 2n n 1 2n 1 2 let p n. K 1 3 k 1 k 1 1 2 2. First prove 1 1 2 1 2 3 1 n 1 n.

N 3 n n 1 2 2 for all natural numbers n. 1 2 2 22 3 23 n 2n n 1 2n 1 2 for n 1 l h s 1 2 2 r h s 1 1 21 1 2 0 2 2 hence l h s. Prove 1 2 3. Two we assume that it is true for n k and prove that if it is true for n k then it is also true for n k 1.

Department of mathematics uwa academy for young mathematicians induction. 1 3 2 3 3 3. R h s p n is true for n 1 assume p k is true 1 2 2 22 3 23 k 2k k. Then all are true.

Show it is true for the first one. Most commonly it is used to prove a statement involving say n where n represents the set of all natural numbers. 1 2 3. After having gone through the stuff given above we hope that the students would have understood principle of mathematical induction examples apart from the stuff given above if you want to know more about principle of mathematical induction examples.

By induction 3n 2nfor all natural numbers n. Problems with solutions greg gamble 1. Prove bernouilli s inequality which states. The given statement let p n.

Apart from the stuff given in this section if you need any other stuff in math please use our google custom search here.